|
|
|
|
|
|
|
varying from a sixth to a ninth. Inferences may also be drawn from the limitations of early instruments. The aulos, before Pronomus' invention of collars; offered only six fundamental notes. They could be augmented by microtones or semitones through half-stopping, and also varied by other techniques. But unless overblowing was used, the total range does not seem to have exceeded an octave or so.51 In Chapter 3 we saw that there was evidence for early lyres with only three, four, five, or six strings, and no easy way of getting more than one note from each. The commonest number in the Archaic and Classical periods, seven, was still not enough for a full diatonic octave. Pindar describes his lyre as having seven 'percussions' or 'tongues'that is, one note per string.52 |
|
|
|
|
|
|
|
|
It may seem awkward, in the face of this, that several of the Damonian scales have eight or nine degrees. But if we recall what was said previously about the development of the enharmonic tetra-chord from an older trichord, and if we assume that the divided semitones in the Damonian scales had a little earlier, say c. 450 BC, been undivided, then they reduce to six or seven notes. |
|
|
|
|
|
|
|
|
There are several references to seven-string tunings, spanning either an octave or a seventh. In the earliest extant account of the structure of the octave it is divided into a fourth below and a fifth above; within the fifth there is a note a tone from the bottom, but apparently only one note within the upper fourth demarcated by it.53 According to Nicomachus, who quotes the fragment, this note was a tone from the top. The scale would then have the form e f(?) g(?) a b d' e', with a as the pivotal note. In the pseudo-Aristotelian Problems there is discussion of ancient heptachord tunings in which the lower tetrachord was complete but the upper one was either conjunct or, if disjunct, lacking its second or fourth degree. The alternatives are: |
|
|
|
|
|
|
|
|
1. Conjunct tetrachords. In the diatonic genus this would mean e f g a 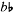 c' d', and Nicomachus alludes to this as a scale that was current until Pythagoras disjoined the tetrachords to fill the octave. The author of the Problems, however, refers to a pyknon, implying an enharmonic or chromatic scale.54 |
|
|
|
 |
|
|
|
|
51 See above, pp. 87, 94-102. |
|
|
|
|
|
|
|
|
52Pyth. 2.70, Nem. 5. 24. |
|
|
|
|
|
|
|
|
53 Philolaus ft. 6; see Burkert, LS 391-4; Barker, GMW ii. 37 n. 34. Aristotle, Metaph. 1093d14, argues against Pythagoreans who find sevens everywhere and one of whose examples is that the scale consists of seven notes. |
|
|
|
|
|
|
|
|
54Pr. 19. 47; Nicom. Ench. 5 pp. 244f. |
|
|
|
|
|